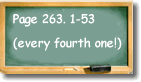| ADVANCED FUNCTIONS: ESSENTIALS OF PRECALCULUS (BABYLONIAN v EUCLIDEAN METHODS) | |||||
| 5. APPLICATIONS OF TRIGONOMETRY AND TRIGONOMETRIC IDENTITIES | |||||
|---|---|---|---|---|---|
| CLASS | TEXT | KNOWLEDGE & APPLICATION | THINKING & COMMUNICATION | HOMEWORK | ENRICHMENT |
| FINAL EXAM
- Monday June 2. 12:30 pm in the Gym |
|||||
75 May 26 |
354 458 |
Chapter 4 & 5 Review |
|||
74 May 22 |
198 286 |
Chapter 2 & 3 Review |
|||
73 May 20 |
418-421 |
5.5 Trigonometric Equations Take
up Homework |
|||
72 May 15 |
418-421 |
5.5 Trigonometric Equations
|
 |
||
71 May 13 |
414-417 |
5.5 Trigonometric Equations
|
 |
||
70 May 9 |
409-410 |
5.4 Inverse Trigonometric Equations III
|
 |
||
69 May 7 |
408-409 |
5.4 Inverse Trigonometric Functions II
ASSESSMENT 7 |
 |
||
68 May 5 |
404-408 |
5.4 Inverse Trigonometric Functions I
|
 |
||
67 May 1 |
402-404 |
5.4 Inverse Trigonometric Functions
|
 |
||
66 Apr 29 |
388-394 |
5.3 More on Trigonometric Identities
I
|
 |
||
65 Apr 25 |
381-384 |
5.2 Verification of Trigonometric Identities III
|
 |
||
64 Apr 23 |
378-381 |
5.2 Verification of Trigonometric Identities II
|
 |
||
63 Apr 18 |
375-378 |
5.2 Verification of Trigonometric Identities I
|
 |
||
62 Apr 16 |
371-374 |
Section 5.1. Trigonometric Functions of Acute Angles
|
 |
||
61 Apr 14 |
367-370 |
Section 5.1. Trigonometric Functions of Acute Angles
|
 |
||
60 Apr 10 |
359-363 |
|
 |
||
59 Apr 8 |
|
 |
|||
4. TRIGONOMETRIC (CIRCULAR) FUNCTIONS |
|||||
CLASS |
TEXT |
KNOWLEDGE & APPLICATION | THINKING & COMMUNICATION |
HOMEWORK | ENRICHMENT |
58 Apr 4 |
342-346 |
Section 4.5: Graphing Techniques:
|
 |
||
57 Apr 2 |
Section 4.5: Graphing Techniques:
|
 |
|||
56 Mar 31 |
|
 |
|||
55 Mar 27 |
331-338 |
4.4 Graphs of the Other Trigonometric Functions
|
|
|
|
MARCH BREAK |
|||||
54 Mar 7 |
331-338 |
4.4 Graphs of the Other Trigonometric Functions
|
|
|
|
53 Mar 5 |
Transformational Graphing
|
|
|
||
53 Mar 3 |
329-330- |
Take Up Exercise Set 4.3
|
|
|
|
52 Feb 28 |
322-328 |
|
|
||
51 Feb 26 |
313-318 |
4.2 Trigonometric (Circular) Functions of Real Numbers
|
|
|
|
50 Feb 21 |
313-318 |
4.2 Trigonometric (Circular) Functions of Real Numbers
|
|
|
|
49 Feb 19 |
307-313 |
4.2 Trigonometric (Circular) Functions of Real Numbers
|
|
|
|
48 Feb 15 |
307 |
4.2 Trigonometric Functions of Real Numbers
|
Manipula Math Applets |
|
|
47 Feb 13 |
296-302 |
4.1 A3: Angles, Arcs and Areas
|
|
|
|
46 Feb 11 |
291-296 |
Return and Review Assessment 5
|
Determine the ratio of the area of a circle to it's inscribed square.
|
|
|
3. EXPONENTIAL AND LOGARITHMIC FUNCTIONS |
|||||
CLASS |
TEXT |
KNOWLEDGE & APPLICATION | THINKING & COMMUNICATION |
HOMEWORK | ENRICHMENT |
45 Feb 7 |
3.6 Exponential Growth and Decay
|
|
|
|
|
44 Feb 5 |
Assessment 5 |
|
|
|
|
43 Jan 30 |
284-285 |
3.5 Exponential and Logarithmic Applications Chapter 3 True/False Exercises Chapter 3 Review Questions |
|
|
|
42 Jan 28 |
257-266 |
3.5 Exponential and
Logarithmic Equations
|
As we recapped in Lesson 40's T&C section, the log of a sum is not the sum of the logs. However, for some combinations
it is true. Solve for x in terms of y for the following equation:
|
|
|
41 Jan 24 |
245-251 |
3.4 Logarithms and Logarithmic
Scales
|
Solve for x:
|
|
|
40 Jan 22 |
243-245 |
3.4 Logarithms and Logarithmic
Scales
|
(Tricky) Is
|
|
|
39 Jan 18 |
230-238 |
3.3 Logarithmic Functions and Their Applications
|
|
|
|
38 Jan 16 |
Chapter
2 Test |
|
|
|
|
37 Jan 14 |
230-238 |
Take Up Homework: 224-226 3.3 Logarithmic Functions and Their Applications
|
|
|
|
36 Jan 10 |
215-224 |
Take Up Homework: 212-213
|
On a single Cartesian Plane, draw the graphs of f(x)=ex and g(x)=e-x. Now, overlay the graphs of |
224-226: |
|
35 Jan 8 |
215-226 |
Simplify the expression,
|
224-226:
|
||
34 Dec 18 |
196-197 |
|
196-197: |
|
|
33 Dec 12 |
203-214 |
Section 3.1. Inverse Functions
|
Can a function be its own inverse? |
212-213: |
|
2. POLYNOMIAL AND RATIONAL FUNCTIONS |
|||||
CLASS |
TEXT |
KNOWLEDGE & APPLICATION | THINKING & COMMUNICATION |
HOMEWORK |
ENRICHMENT |
32 Dec 10 |
177-192 |
Section 2.6. Graphs
of Rational Functions and Their Application
|
Can a function cross its horizontal asymptote? |
189: |
|
31 Dec 6 |
177-192 |
Section 2.6. Graphs of Rational Functions
and Their Applications
|
Can a function have more than one vertical asymptote? Can a function have more than one horizontal asymptote? |
189: |
|
30 Dec 4 |
177-192 |
Section 2.6. Graphs of Rational Functions
and Their Applications
|
Can a function cross it's vertical asymptote? How about its horizontal asymptote? |
189: |
|
29 Nov 30 |
154-167 |
Section 2.4. Zeros of Polynomial Functions
|
|
164-165: |
Double Wheel: Mystery Uncovered |
28 Nov 28 |
138-153 |
Section 2.3. Polynomial Functions of Higher
Degree
|
|
149-151: 1-49 (odd) |
|
27 Nov 26 |
Assessment #3 (1 hour): Sections 1.6, 1.7, and 2.2 |
For the polynomial P(x), if |
| ||
26 Nov 22 |
138-153 |
Section 2.3. Polynomial Functions of Higher
Degree
|
Watch this animation and then determine how much area is 'under' the function, f(x)=x3 over the same closed interval, [1,4].
|
149-151: 1-49 (odd) |
|
25 Nov 20 |
127-137 |
Section 2.2. The Remainder Theorem and the
Factor Theorem
|
136-137: 55, 57, 59, 65, 67 |
(an+bn) |
|
24 Nov 16 |
127-137 |
Section 2.2. The Remainder Theorem and the
Factor Theorem
|
What is a Polynomial in x? |
135-136: 1-54 (every fourth) |
|
23 Nov 14 |
Detailed Review of Section 1.7 | Consider the complex number a+bi. Its complex conjugate
is a-bi. Is the the product of a complex number and its conjugate, real or complex? |
|||
1. FUNCTIONS AND GRAPHS |
|||||
CLASS |
TEXT |
KNOWLEDGE & APPLICATION | THINKING & COMMUNICATION |
HOMEWORK |
ENRICHMENT |
22 Nov 8 |
99-104 |
Section 1.7. The Algebra of Functions
|
105-106: 65-71 (odd) Confirm answers at back |
|
|
21 Nov 6 |
96-99 |
Section 1.7. The Algebra of Functions
|
What are the real solutions (x-intercepts) of the quotient function: (f/g)(x)? |
104-105: 1-64 (every fourth) |
(an-bn) |
20 Nov 2 |
86-90 |
Section 1.6. Properties of Graphs
|
Which of the following geometric transformations does NOT modify the
real solutions of f(x)?
|
91-92: 1-67 (odd) Confirm answers at back |
(p+q)n |
19 Oct 31 |
|
What transformation of f(x) is defined by -f(-x)?
|
91-92: 1-67 (odd) Confirm answers at back |
||
18 Oct 29 |
81-86 |
|
What are the necessary conditions for the maximum value
of f(x)
=
ax2+bx+c
|
91-92: 1-67 (odd) Confirm answers at back |
FOUNDATIONS FOR THE BINOMIAL THEOREM: Factorial Notation Combinations Pascal's Triangle |
17 Oct 25 |
71-74 |
Reliable Steps for Solving Applications
|
Are cubes and cylinders examples of prisms? |
76-77: 45-55 (odd) Confirm answers at back |
DESIGNER |
16 Oct 23 |
67-71 |
|
Is the area enclosed by the x-axis, the line x=1,
and the parabola defined by f(x)=x2 more or less than
1/2 unit2 ? |
75-76: 1-43 (odd) Confirm answers at back |
|
15 Oct 19 |
59-65 |
|
The slope of a line is a well-understood concept. Can a meaning be attached
to the concept of the slope of a curve? |
61-65: 51, 53, 62, 65, 67, 71, 73, 75, 77, 79 Confirm answers at back |
|
14 Oct 17 |
56-59 |
|
Express the previous night's homework question numbers as a
linear function, Q(w), where w is a whole number. |
61-65: 51, 53, 62, 65, 67, 71, 73, 75, 77, 79 Confirm answers at back |
|
13 Oct 15 |
51-56 |
|
What is the two-intercept form of a linear relation?
|
60-61: 1-49 (every sixth) Confirm answers at back |
|
12 Oct 11 |
Assessment 2: Sections 1.2 and 1.3 |
True or False? The square of a sum is equal to the sum of the squares. |
|||
1 Oct 9 |
37-43 |
|
True or False? |
44-45: 43-63 (odd) Confirm answers at back |
|
10 Oct 4 |
30-36 |
|
If f(a)=f(b) does that necessarily mean that
a=b? |
making good use of your elastic! |
|
9 Oct 2 |
30-36 |
|
If a collar tied snugly around the equator of the earth had an
extra metre added to its length, would a mouse be able to crawl underneath
it? |
43-44: 1-41 (odd) Confirm answers at back making good use of your elastic! |
|
8 Sep 28 |
26-27 |
|
Beginning at the eastern most point on a circle, how many radial
lengths, r, could you string around the circle before returning
to the starting point? |
28: 57-75 (odd) Confirm answers at back |
|
7 Sep 26 |
19-26 |
|
Is the reciprocal of x always less than x? |
28: 27-55 (odd) Confirm answers at back |
|
6 Sep 24 |
16-19 |
|
If two mutually perpendicular axes divide R2 into
4 quadrants,
three mutually axes divide R3 into 8 what? |
27-28: 1-23 (odd) Confirm answers at back |
|
5 Sep 19 |
3-12 17-18 |
|
CF asks: "Which set of numbers is more
numerous, the Naturals or the Reals?" |
27-28: 1-23 (odd) Confirm answers at back |
|
4 Sep 17 |
5-12 |
|
Is the square root of a positive real number, x,
always less than x? |
13: 19-57 odd Confirm answers at back |
|
3 Sep 13 |
4-5 |
|
What real number is equal to its reciprocal? Can you name any others? |
13: 19-57 odd Confirm answers at back |
|
2 Sep 11 |
3-4 |
|
What is the first positive real
number? |
13:
1, 5, 9, 13, 17, 59-75 (odd) and graph each interval Confirm answers at back |
|
1 Sep 7 |
3 |
Course of Study, Subject, Forum Textbook and calculator distribution The Hierarchy of the Real Numbers |
Are there more rational numbers
or irrational numbers? |
13:
1, 5, 9, 13, 17, 59-75 (odd) and graph each interval Confirm answers at back |
|
| START OF COURSE | |||||